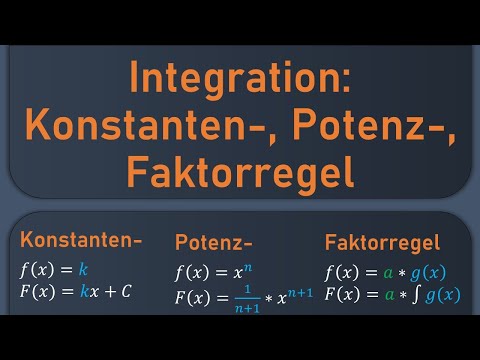
Konstanten, Potenz- und Faktorregel

Tipp

Vergiss nicht bei unbestimmten Integralen spätestens am Ende noch die Integrationskonstante $\color{purple}{C}$ zu setzen.

Konstantenregel

Wenn im Integral nur eine Konstante $k$ steht, dann ist das Integral:

$\int k\,\mathrm{d}x=kx+C$

Beispiele

$\int \color{red}{2}\,\mathrm{d}x=\color{red}{2}x\color{purple}{+C}$
$\int \color{red}{5}\,\mathrm{d}x=\color{red}{5}x\color{purple}{+C}$

Potenzregel

Das Integral von Potenzen in der Form $x^n$ ist:

$\int x^n \,\mathrm{d}x=$ $\frac{1}{n+1}x^{n+1}+C$

Beachte

$n$ darf jede beliebige reelle Zahl außer -1 sein, denn sonst würde man durch 0 dividieren.

$n\in\mathbb{R}\backslash\{-1\}$

Beispiele

$\int x^\color{red}{3} \, \mathrm{d}x $ $= \frac{1}{\color{red}{3}+1}x^{\color{red}{3}+1} $ $= \frac{1}{4}x^{4}\color{purple}{+C}$

$\int x^\color{red}{-2} \, \mathrm{d}x $ $= \frac{1}{\color{red}{-2}+1}x^{\color{red}{-2}+1} $ $= -x^{-1} \color{purple}{+C}$

Faktorregel

Ein konstanter Faktor kann vor das Integralzeichen gezogen werden.

$\int a \cdot g(x) \, \mathrm{d}x =$ $ a \cdot \int g(x) \, \mathrm{d}x$

Beispiele

Hier wird die Potenzregel und die Faktorregel angewendet:

$\int\color{red}{4}x^3 \, \mathrm{d}x$ $=\color{red}{4}\cdot \int x^\color{blue}{3} \,\mathrm{d}x$ $=4\cdot\frac{1}{\color{blue}{3}+1}x^{\color{blue}{3}+1}$ $=x^4\color{purple}{+C}$

$\int\color{red}{-3}x \, \mathrm{d}x$ $=\color{red}{-3}\cdot \int x^\color{blue}{1} \,\mathrm{d}x$ $=-3\cdot\frac{1}{\color{blue}{1}+1}x^{\color{blue}{1}+1}$ $=-\frac32x^2\color{purple}{+C}$

Beachte

Es ist immer wichtig zu beachten, nach welcher Variable integriert wird.

Beispiel:
$\int 3\,\color{red}{\mathrm{d}y}=3\color{red}{y}+C$

Konstanten- Potenz- Faktorregel beim Integrieren, Integrationsregeln, Stammfunktion

Kooperation mit dem Kanal von Mister Mathe

Die Konstantenregel, die Potenzregel und die Faktorregel sind die drei grundlegendsten Regeln zum Integrieren von Funktionen.

Tipp

Wenn man die Ableitungsregeln gut beherrscht, kann man diese auf sein Ergebnis anwenden. Wenn die Ableitung des Ergebnisses die Ausgangsfunktion ergibt, dann wurde richtig integriert.